Input Y Range is where the response variable (Sales in our case) is located. If you’re using the CSV or XSLX file, you should mirror these options. Now that we can select different built-in analyses, we’ll launch the regression tool. You’ll then select the Analysis Toolpak and it should now be visible in the Data tab. Select the Add-ins section and go to Manage Excel Add-ins. 
Go to the Data tab, right-click and select Customize the Ribbon. If you don’t have the Toolpak (seen in the Data tab under the Analysis section), you may need to add the tool. Y = 1,383.471380 + 10.62219546 * X Doing Simple and Multiple Regression with Excel’s Data Analysis ToolsĮxcel makes it very easy to do linear regression using the Data Analytis Toolpak. We now have our simple linear regression equation. The intercept is the “extra” that the model needs to make up for the average case. To calculate our regression coefficient we divide the covariance of X and Y (SSxy) by the variance in X (SSxx) This is a video presented by Alissa Grant-Walker on how to calculate the coefficient of determination.The sum fields are our SSxx and SSxy (respectively). For more information, please see [ Video Examples Example 1 
To account for this, an adjusted version of the coefficient of determination is sometimes used. Thus, in the example above, if we added another variable measuring mean height of lecturers, $R^2$ would be no lower and may well, by chance, be greater - even though this is unlikely to be an improvement in the model. This means that the number of lectures per day account for $89.5$% of the variation in the hours people spend at university per day.Īn odd property of $R^2$ is that it is increasing with the number of variables. There are a number of variants (see comment below) the one presented here is widely used
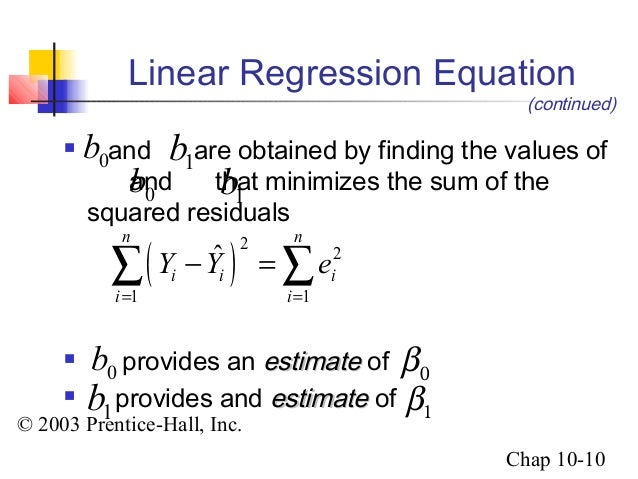
It is therefore important when a statistical model is used either to predict future outcomes or in the testing of hypotheses. In the context of regression it is a statistical measure of how well the regression line approximates the actual data. The coefficient of determination, or $R^2$, is a measure that provides information about the goodness of fit of a model.
Contents Toggle Main Menu 1 Definition 2 Interpretation of the $R^2$ value 3 Worked Example 4 Video Examples 5 External Resources 6 See Also Definition
0 kommentar(er)
